

 字體:小 中 大
字體:小 中 大 |
|
|
|
| 2021/02/14 16:19:41瀏覽5638|回應0|推薦0 | |
Chap. 2 Reciprocal lattice crystal structure: basis + lattice bobe beam for studying crystal structure: 1. x rays 光子與electron作用, =1-10Å. 2. neutron nuclei. 3. electron Bragg’s law 2dsin=n 加強性干涉
n(r) electron concentration charge density, r=r+T 滿足週期性關係 satisfied n(r+T )=n(r)=n( r) → n( r)≡periodic, T =u₁a₁+u₂a₂+u₃a₃ consider 1-D n(x+a)=n(x), T =u₁a₁, n(x) can be represented by a Fourier series
n(x)=n₀+∑ₚ>₀[Cpcos(2πpx/a)+Spsin(2πpx/a)] n(x+a)=n₀+∑ₚ>₀[Cpcos(2πpx/a+2πp)+Spsin(2πpx/a+2πp)]=n(x) n(x)=n₀+∑ₚ>₀[Cp(ei2πpx/a+e-i2πpx/a)/2-iSp(ei2πpx/a-e-i2πpx/a)/2] =n₀+∑ₚ>₀[ei2πpx/a(Cp-iSp)/2+e-i2πpx/a(Cp+iSp)/2] =∑pnp ei2πpx/a Cp, Sp are real, n-p=np* n-p=½( Cp-iSp), np*=½( Cp+iSp) n(x)=∑pnp ei2πpx/a → 2π/a is 1-D reciprocal lattice vector. In 3-D, we have to define a vector G and satisfy n(r+T )=n(r)=n(r) n(r)=∑G nGeiG•r 倒空間平移向量: G=v₁b₁+v₂b₂+v₃b₃, b₁=2π[(a₂a₃)/(a₁•a₂a₃)], b₂=2π[(a₃a₁)/(a₁•a₂a₃)], b₃=2π[(a₁a₂)/(a₁•a₂a₃)] i.e. V= a₁•a₂a₃ b₁•a₁=2π , b₁•a₂=0, b₁•a₃=0. If a₁, a₂, a₃ are primitive axes, then b₁, b₂, b₃ are primitive axes. bᵢ•aj=2πδᵢj, δᵢj=1(i=j) or δᵢj=0(i≠j) → reciprocal lattice used in x ray diffraction n(r+T )=∑G nGeiG•(r +T)=∑G nGeiG•r ∑G nGeiG• T=n(r)ei2π(v₁u₁+v₂u₂+v₃u₃)=n(r)
the amplitude of an incident plane wave at r is A=A₀ei(k•r-wt) at site 1, amplitude is A=A₀e-iwt at site 2, amplitude is A=A₀ei(k•ρ-wt) x ray scattered from #1 electron, amplitude =2k•G=|G|² Step 1. if G=hb₁+kb₂ +lb₃ then G is normal to the crystal plane (h,k,l)
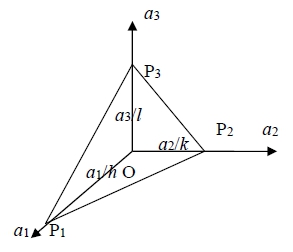
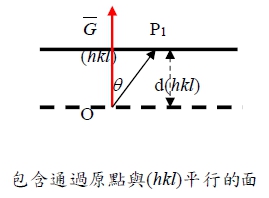
找平面的法線方向, n=P₁P₂P₁P₃=(a₂/k-a₁/h)(a₃/l-a₁/h)=1/kl(a₂a₃)-1/hl(a₁a₃)-1/hk(a₂a₁) n=1/kl(a₂a₃)-1/hl(a₁a₃)-1/hk(a₂a₁)=(1/hkl)[h(a₂a₃)+k(a₃a₁)+l(a₁a₂)] =((a₁•a₂a₃)/2πhkl){2π[h(a₁a₂)/(a₁•a₂a₃)]+2π[k(a₃a₁)/(a₁•a₂a₃)]+2π[l(a₂a₃)/(a₁•a₂a₃)]} =((a₁•a₂a₃)/2πhkl)[hb₁+kb₂ +lb₃]=(V/2πhkl) G // G ⸪ n // G ⸫ G ┴ (hkl) Step 2. 平面間距離 d(hkl)=2π/|G|
包含通過原點與(hkl)平行的面, OP₁•G=|OP₁||G|cosθ → d(hkl)=|OP₁|cosθ=(OP₁•G)/|G|= {(a₁/h)•[hb₁+kb₂ +lb₃]}/|G|=2π/|G|
Step 3. 2k•G=|G|² k+G=k → k-k=G i.e. |k|=|k| k²=k•k=|k|²=(k+G)•(k+G)=|k|²+2k•G+|G|² → 2k•G+|G|²=0 → 2k•(-G)+|-G|²=0 → 2k•G=|G|²
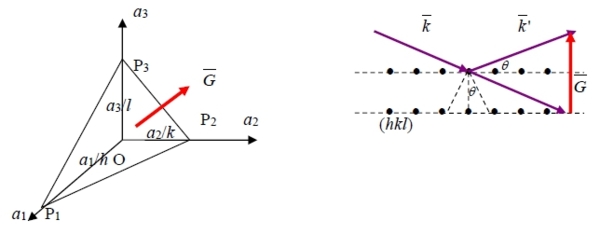
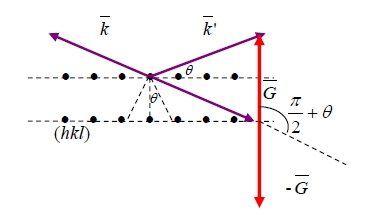
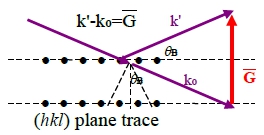
2|k||G|cos(90°+θ)+|G|²=0 → -2|k||G|sinθ+|G|²=0 → 2|k|sinθ=|G|=2πn/d(hkl) → 2(2π/λ)sinθ=2πn/d(hkl) ⸫ 2d(hkl) sinθ=nλ another statement of Bragg’s law Laue equation: 找k的方向, k-k=Δk =G if G=v₁b₁+v₂b₂+v₃b₃ → a₁•Δk =a₁•G=2πv₁, a₂•Δk =2πv₂, a₃•Δk =2πv₃ Edwald construction Brillouin zones: is defined as a wigner-seitz primitive cell in the reciprocal lattice. Diffraction condition: 2k•G=|G|²除以4, k•(G/2)=|G/2|²
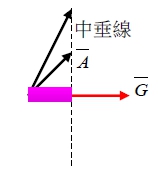
A•(G/2)=|A||G/2|cosθ=|A|cosθ|G/2|=|G/2|² G₁⊥原子平面跡 (plane trace), 原子平面跡是k₀及k的分角線1/2|G|=|k₀|sinθᴃ , |k₀|=1/λ, λ=2/|G|sin θᴃ, 與λ=2d sin θᴃ 比較, |G|=1/d ,且G //原子平面之法向量(有建設性干涉的原子平面⊥原子平面跡 {G}代表晶體可產生“建設性”干涉的平面組,其方向為平行平面之法向量且長度為對應的平面間距
實空間與倒空間(晶體結構與繞射花樣 (Band Gap)為何需要另一個空間? •倒空間能使我們方便描述及處理晶體繞射的問題 •事實上,“倒”晶格空間和“實”晶格空間一樣的“真實” •我們可以把晶體想成有兩個“晶格”一個“實”晶格,一個“倒”晶格實晶格是晶體本身,倒晶格則是繞射空間的點陣 1) 倒空間: 與實空間一樣真實 2) 倒空間的一點相對於實空間的一平面 3)倒空間的一點至原點的距離等於平面間距的倒數
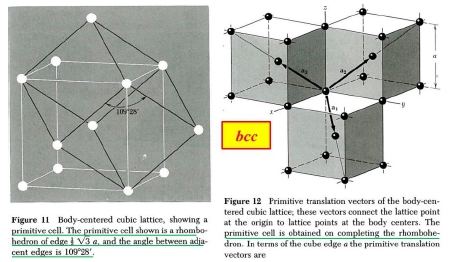
Real space vs. Reciprocal space 實空間平移向量,r=n₁t₁+n₂t₂+n₃t₃, primitive cell是晶體中只含一個原子的unit cell, 以primitive unit cell為單位取實空間平移向量, e.g. BCC t₁=a/2(-x+y+z), t₂=a/2(x-y+z), t₃=a/2(x+y-z)
b₁=2π[(t₂t₃)/(t₁•t₂t₃)], b₂=2π[(t₃t₁)/(t₁•t₂t₃)], b₃=2π[(t₁t₂)/(t₁•t₂t₃)] i.e. V= t₁•t₂t₃ → b₁=2π[(a²/4)(2y+2z)]/(a³/2)=2π/a(y+z)=2π/a(011), b₂=2π/a(101), b₃=2π/a(110)
crystal lattice: a₁=ax, a₂=ay, a₃=az V=a₁∙a₂a₃=a³ reciprocal lattice: b₁=(2π/a)x, b₂=(2π/a)y, b₃=(2π/a)z V=b₁∙b₂b₃=(2π/a)³ remind**G=v₁b₁+v₂b₂+v₃b₃, b₁=2π(a₂a₃/a₁∙a₂a₃), b₂=2π(a₃a₁/a₁∙a₂a₃), b₃=2π(a₁a₂/a₁∙a₂a₃) b₁∙a₁=2π, b₁∙a₂=0, b₁∙a₃=0 第一不連續的cubic邊長=2/a the boundary of the 1st Brillouin zone: the planes normal to the six reciprocal lattice vectors: ±½b₁=±(π/a)x, ±½b₂=±(π/a)y, ±½b₃=±(π/a)z
crystal lattice: a₁=a/2(-x+y+z), a₂=a/2(x-y+z), a₃=a/2(x+y-z) V=|a₁∙a₂a₃| =(a/2)³ reciprocal lattice: b₁=(2π/a)(y+z), b₂=(2π/a)(x+z), b₃=(2π/a)(x+y) V=b₁∙b₂b₃=(2π/a)³ →B.C.C. 的reciprocal lattice = F.C.C.的晶格 the boundary of the 1st Brillouin zone: the planes normal to the twelve reciprocal lattice vectors: b₁=(π/a)(±y±z), b₂=(π/a)(±x±z), b₃=(π/a)(±x±y)
crystal lattice: a₁=a/2(y+z), a₂=a/2(x+z), a₃=a/2(x+y) V=|a₁∙a₂a₃|=(a/2)³ reciprocal lattice: b₁=(2π/a)(-x+y+z), b₂=(2π/a)(x-y+z), b₃=(2π/a)(x+y-z) →F.C.C. 的reciprocal lattice = B.C.C.的晶格 第一不連續的 8 vectors: (π/a)(±x±y±z) Fourier analysis of the basis Consider one cell, the amplitude of x ray scatter from a crystal of N cells can be written as: FG=N∫сеӏӏdVn(r)e-i∆k∙r=N∫сеӏӏdVn(r)e-iG ∙r=N∙SG structure factor, SG=∫сеӏӏdVn(r)e-iG ∙r, 分成atom, electron兩邊討論
SG=∫сеӏӏdVn(r)e-iG ∙r=∫сеӏӏdV∑j nj(r-rj)e-iG ∙r=∑j ∫сеӏӏdVnj(r-rj)e-iG ∙r , let ρ=r-rj →SG=∑j ∫сеӏӏdVnj(ρ)e-iG ∙r 在積分號rj 為常數 SG=∑j ∫сеӏӏdVnj(ρ)e-iG ∙(ρ+ rj)=∑j ∫сеӏӏdVnj(ρ)e-iG ∙ρ e-iG ∙rj=∑j e-iG ∙rj∫сеӏӏdVnj(ρ)e-iG ∙ρ =∑j e-iG ∙rj∙fj fj: 考慮何種atom對電子的影響, we called it atom form factor. 首項級數和: 是考慮atom的位置, 亦即晶體結構的影響 考慮basis中的atom位置 rj=xja₁+yja₂+zja₃ 0≤xj, yj, zj≤1 G=v₁b₁+v₂b₂+v₃b₃, bᵢ∙aj=2πδᵢj, δᵢj=0(i≠j) or δᵢj=1(i=j) G ∙rj=2π(v₁xj+v₂yj+v₃zj) → SG=∑j e-i2π(v₁xj+v₂yj+v₃zj)∙fj
basis = one atom (0,0,0) → SG=fje-i2π(0)=f any set of (v1,v2,v3) can satisfy the constructive diffraction condition.
conventional cell: positions of atoms→ (0,0,0), (½,½,½) SG=∑j e-i2π(v₁xj+v₂yj+v₃zj)∙fj=f∙e-i2π(0)+f∙e-iπ(v₁+v₂+v₃)=f∙[1+e-iπ(v₁+v₂+v₃)] e-iθ=cosθ-isinθ, θ=nπ if v₁+v₂+v₃=2n, SG=2f (constructive) or v1+v2+v3=2n+1, SG=0 (destructive)
positions of atoms→ (0,0,0), (½,0,½), (½,½,0), (0,½,½) SG=f∙[1+e-iπ(v₂+v₃)+e-iπ(v₁+v₃)+e-iπ(v₁+v₂)] if (i)v₁+v₂+v₃=2n, SG=4f (constructive) (ii)v1+v2+v3=2n+1, SG=4f (constructive) (iii)1odd, 2even → SG=0 (destructive) (iv)2odd, 1even → SG=0 (destructive) ex. Structure factor of KCl position of atom in a cell: Cl- : (0,0,0), (½,0,½), (½,½,0), (0,½,½) K+ : (½,½,½), (0,0,½), (0,½,0), (½,0,0) SG=f(K⁺)[e-iπ(v₁+v₂+v₃)+e-iπv₁+e-iπv₂+e-iπv₃]+f(Cl⁻)[1+e-iπ(v₂+v₃)+e-iπ(v₁+v₃)+e-iπ(v₁+v₂)] if (i)v₁+v₂+v₃=2n, SG=4[f(K⁺)+f(Cl⁻)] (constructive) (ii)v1+v2+v3=2n+1, SG=4f[f(Cl⁻)-f(K⁺)]≈0 (⸪Cl⁻≈K⁺) (iii)1odd, 2even → SG=0 (destructive) (iv)2odd, 1even → SG=0 (destructive) p.s. KBr → f(Br⁻)≠f(K⁺) ⸫SG=4[f(K⁺)-f(Br⁻)]≠0 Summary FG=N∫сеӏӏdVn(r)e-i∆k∙r=N∫сеӏӏdVn(r)e-iG ∙r=N∙SG SG=∑j ∫сеӏӏdVnj(ρ)e-iG ∙(ρ+ rj)=∑j ∫сеӏӏdVnj(ρ)e-iG ∙ρ e-iG ∙rj=∑j e-iG ∙rj∫сеӏӏdVnj(ρ)e-iG ∙ρ =∑j e-iG ∙rj∙fj fj = atom form factor scattered radiation from a single atom fj =∫сеӏӏdVnj(ρ)e-iG ∙ρ k-k=∆k=G |k|=|k| ⸫|G|=2|k|sinθ =2(2π/λ)sinθ =(4π/λ)sinθ → f(|G|)=f(sinθ/λ)
consider a spherical distribution n(r)=n(r,,)=n(r)只隨r改變, ,改變均不變(球面上密度都一樣) dV=r²sindrdd fj =∫∫∫r²sindrdd[n(r)e-iG ∙r]=∫r²dr∫sin d ∫d n(r)e-i|G|∙|r|cosθ =2π∫r²n(r)dr∫sin∙e-iGrcosθd P.S. ∫sin∙e-iGrcosθd=∫∙e-iGrcosθd(-cos)=e-iGrcosθ/iGr|π0=(eiGr-e-iGr)/iGr=2sin(Gr)/Gr → fj =4π∫[r²n(r)sin(Gr)/Gr]dr if z(electron density)集中在r=0處, n(r)=zδ(r), limit δ(x)=1/τ, 0<x<τ or =0, |x|>τ when τ→0 ⸪ r→0, sin(Gr)/Gr=1 fj =4π∫[r²zδ(r)]dr=∫zδ(r)∙4πr²dr=∫zδ(r)∙dV=z |
|
| ( 知識學習|隨堂筆記 ) |





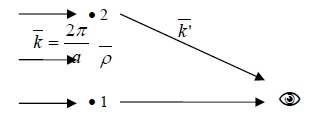

 = a³/2
= a³/2 =2(2π/a)³
=2(2π/a)³ 






